DiogoJ42
TS Member
- Favourite Ride
- The Metropolitan Line
OK, so this has spawned out of a rather metaphysical Skype conversation. Please bear with me.
I assume we are all aware of the concept of a "Möbius" coaster, such as Grand National...
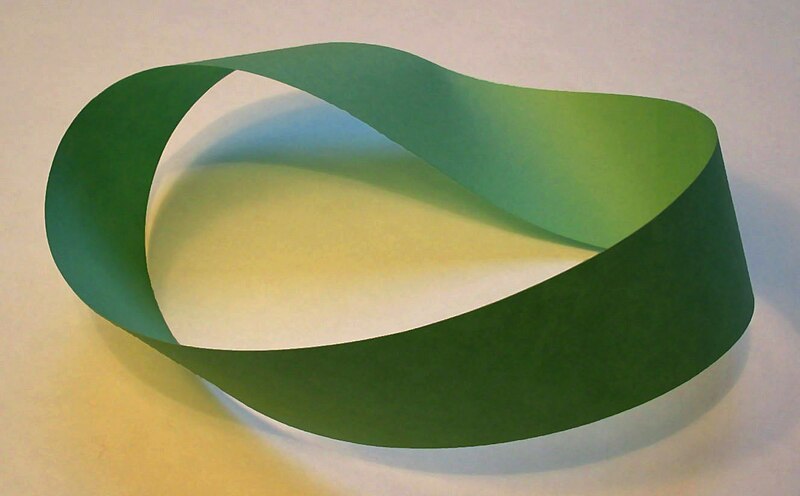
This takes it's name from the "Möbius Strip": a surface with only one side (when embedded in three-dimensional Euclidean space) and only one boundary.
But what if we knocked it up a notch? The three dimensional equivalent to a Möbius Strip is the so-called "Klein Bottle": a two-dimensional manifold against which a system for determining a normal vector cannot be consistently defined. Informally, it is a one-sided surface which, if traveled upon, could be followed back to the point of origin while flipping the traveler upside down. Other related non-orientable objects include the Möbius strip and the real projective plane. Whereas a Möbius strip is a surface with boundary, a Klein bottle has no boundary (for comparison, a sphere is an orientable surface with no boundary).
And it "looks like" this:

SO: if it is possible to have a "Möbius" coaster, then logically, it must be possible to build a "Klien" coaster.
.... The question is, what in the name of Wardley would this involve? Three racing tracks that count as one? Or would it transcend our known universe and start involving the so-called "fourth dimension" of time?
Discuss!
I assume we are all aware of the concept of a "Möbius" coaster, such as Grand National...

This takes it's name from the "Möbius Strip": a surface with only one side (when embedded in three-dimensional Euclidean space) and only one boundary.
But what if we knocked it up a notch? The three dimensional equivalent to a Möbius Strip is the so-called "Klein Bottle": a two-dimensional manifold against which a system for determining a normal vector cannot be consistently defined. Informally, it is a one-sided surface which, if traveled upon, could be followed back to the point of origin while flipping the traveler upside down. Other related non-orientable objects include the Möbius strip and the real projective plane. Whereas a Möbius strip is a surface with boundary, a Klein bottle has no boundary (for comparison, a sphere is an orientable surface with no boundary).
And it "looks like" this:

SO: if it is possible to have a "Möbius" coaster, then logically, it must be possible to build a "Klien" coaster.
.... The question is, what in the name of Wardley would this involve? Three racing tracks that count as one? Or would it transcend our known universe and start involving the so-called "fourth dimension" of time?
Discuss!
Last edited: